Abstract
Prestack depth migration
Seismic imaging has come a long way over the years. It has evolved from its ancient days as a mechanical tool applied to picked data from single-fold reflection records, through its early digital incarnation as poststack wave-equation migration, to its present "mature" state as prestack depth migration. During this time, seismic imaging has grown to encompass more and more of the seismic processing flow. The evolution to its present state, and even beyond, is to be expected: after all, seismic reflection data have always been acquired with the goal of imaging the Earth's interior for oil and gas exploration.
Present-day seismic imaging is at the center of the processing flow, and for some it also occupies a central role in interpretation. Prestack depth migration (PSDM) is used to produce depth maps of prospective horizons, to estimate velocities used in depth conversion, to provide input to AVO and rock property analysis, and, in general, to reduce structural and stratigraphic risk in the process of drilling exploration and development wells. PSDM is used in deep-water areas such as the Gulf of Mexico and the Canadian East Coast, in rugged land areas such as the Canadian Foothills and the jungles and mountains of South America, in permafrost areas such as the Mackenzie Delta. It is used to help find and delineate elephant fields, and it is used to define subtle structural and stratigraphic traps.
PSDM has so many uses for so many play types in so many areas, can it possibly be a single process? Can it even involve a single, unified flow from start to finish? Further, will it work the same on all styles of data acquisition, land and marine, 2-D and 3-D? Unfortunately, no single PSDM flow will work better than all others for all play types and all styles of acquisition. But we can take some comfort in the fact that PSDM solutions (often more than one) exist for each problem.
Kirchhoff migration - The workhorse method
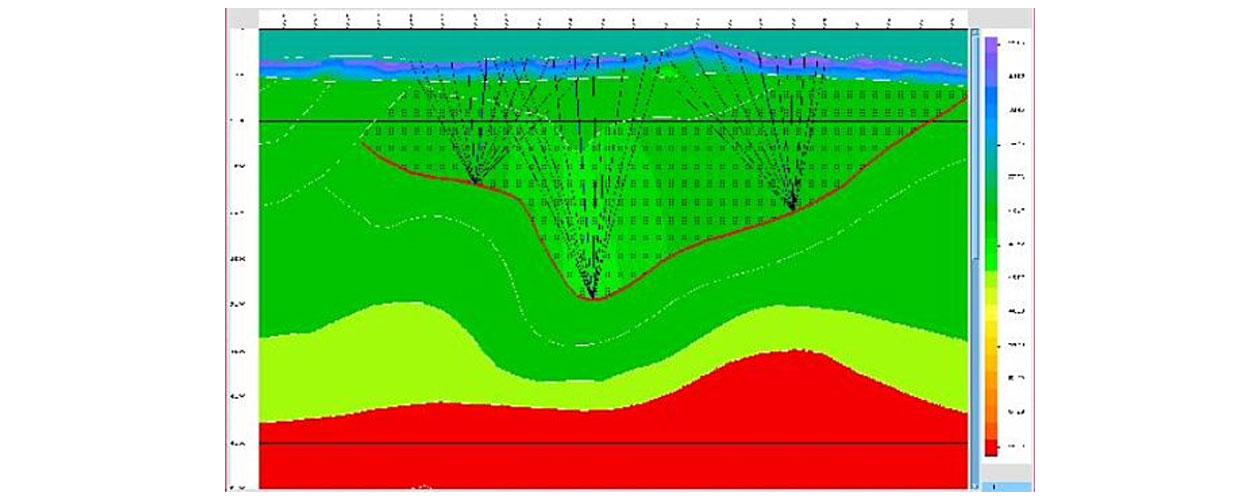
One PSDM solution does fit most problems, even if it is not the best solution for many of them. This is the ubiquitous Kirchhoff migration, which has dominated the imaging landscape for the past decade. Kirchhoff migration is almost infinitely versatile: it can take seismic data from almost any surface and/or subsurface acquisition geometry and produce an image on almost any other subsurface target geometry. Kirchhoff migration programs have been optimized to produce huge 3-D output volumes from even huger 3-D input volumes, and to produce small 2-D targets from crooked lines. Our ability to raytrace through anisotropic layers greatly increases the accuracy of Kirchhoff migration in many areas of the world. Last but not least, Kirchhoff PSDM provides migrated gathers that are naturally suited for tomographic velocity estimation techniques. This method does have its limitations, though. The first is fundamental to all imaging methods, and that is its reliance on both the wave equation and the discrete spatial sampling of recorded wavefields. We sometimes ignore this, abusing its versatility when migrating land data, either ancient-vintage 2-D or even reasonably modern 3-D, that are too poorly sampled for any wave-equation process to work properly. The second limitation is its dependence on rays to simulate wave behavior. Rays are a wonderful conceptual tool that allows us to envision seismic wave propagation (Figure 1), and they are also a wonderful computational tool that allows us to compute seismic wavefields very efficiently. On the other hand, rays do not tell the whole story of wave propagation, and sometimes their failure to tell the whole story can result in an incomplete or noisy seismic image from Kirchhoff migration.
Still, Kirchhoff migration has provided the bulk of our PSDM images and the velocity fields that go with those images. And it will continue to do so, at least for land data, at least until we improve our ability to regularize sparse, irregularly sampled wavefields into volumes suitable for more rigorous migration techniques. These "wave-equation," or wavefield continuation, techniques are based on downward continuing well-sampled wavefields from one depth layer to the next. Our continued reliance on Kirchhoff migration is even surer for data with large static problems, though for the wrong reason. Pervasive static problems almost guarantee less-than-perfect migrated images and velocity models, and the efficiency of Kirchhoff migration relative to wavefield continuation techniques implies that we will use Kirchhoff migration to produce these suboptimal images and velocity fields.
Wavefield continuation methods - Greater accuracy, greater cost, less flexibility
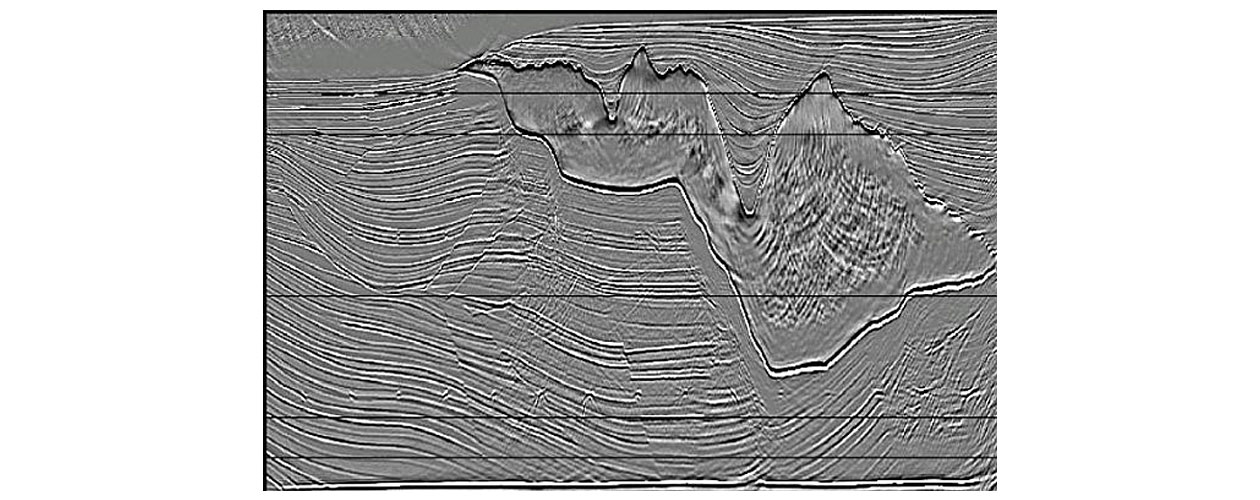
Kirchhoff migration is versatile and usually accurate enough for our imaging problems on land. But where geologic structure is extremely complicated, as it is around salt bodies or carbonate thrust sheets, Kirchhoff migration can fail to produce an accurate image. (This failure might be due to the theoretical inability of the ray-based migration to image accurately in shadow zones or regions of triplication, or it might be due to poor velocity control or even poor implementation. In theory, migration methods can be arranged in a hierarchy of accuracy, with Kirchhoff migration at the bottom and various styles of wavefield continuation methods at the top. But in practice, we should remember that the velocities used in migration, and even the implementation of any of the methods, are as important as the choice of method. In fact, a very good Kirchhoff migration with good velocity control can often produce a more accurate image than a poor implementation of a "wave-equation" migration.) Its theoretical limitations tell us that some geologic structures require greater imaging accuracy than Kirchhoff migration can reasonably provide. Given this fact, we need to pursue more accurate imaging alternatives. For marine streamer records, which are well sampled spatially, wavefield continuation such as finite-difference or screen methods are now a viable alternative to Kirchhoff migration (Figure 2). The recent growth in popularity of these methods is due, in large part, to our ability to assign large clusters of computers, numbering in the thousands of individual nodes, to the largest imaging problems.
The more powerful wavefield continuation migration methods come with their own set of challenges and opportunities for the related problems of velocity estimation and amplitude analysis. Kirchhoff migration is ray-based, and the velocity updating routines that it relies on, ranging from simple vertical updating to traveltime tomography (refraction and reflection), are also ray-based. Wavefield continuation methods don't use rays, so velocity upating routines for these methods should also be wave-based and not ray-based. Some velocity updating routines for Kirchhoff migration are interactive, making them well-suited for interpretive processing. Wavefield continuation methods, however, tend to be used for large marine projects whose scale demands a minimum of human intervention, preferably just a few high-level geological or geophysical choices. So these methods require high-quality, automatic velocity updating routines that don't yet exist as production tools. Further, even the process of assembling migrated gathers, for both migration velocity estimation and amplitude analysis, is fundamentally different for these methods from the process used for Kirchhoff migration. For example, it is easy to index migrated gathers by shot location, somewhat harder to index them by offset (as for Kirchhoff migration), and harder still to index them by subsurface opening angle at the image locations. Although the future of velocity analysis is not certain, it will likely involve some form of automatic full-wave inversion of data from opening-angle gathers. These gathers are also ideal for amplitude analysis, since they automatically provide amplitude-versus-angle (AVA) information, and not just amplitude-versus-offset (AVO) information.
Bridging the gap
The routine application of iterative "wave-equation" migration, with migration velocities coming from a full-wave inversion of opening-angle migrated gathers, and with opening-angle gathers feeding into an AVA analysis, lies in the future. Although the future has a way of sneaking up on us, it is not here yet. Our land surveys are still sparse and irregular. Our largest marine imaging projects still overwhelm our computing capacity. Even as we anticipate the future, we must make intelligent use of today's imaging technology. This means a heavy use of the workhorse Kirchhoff methods for both imaging and velocity estimation, at least in early migration iterations. Gradually, we will begin to introduce wavefield continuation migration into earlier iterations, even as we continue to estimate migration velocities using rays. On land, we will continue to use the versatility of Kirchhoff methods for some time, until we discover some "magic bullet" data regularization process that can transform our data into well sampled wavefields.
Tried-and-true Kirchhoff PSDM, and ray-based velocity estimation methods, will be with us for some time. But eventually we will be able to take advantage of the greater imaging potential of wavefield continuation methods, and we will test the accuracy of velocity and amplitude analysis using opening-angle gathers. The challenge of this next generation of seismic imaging tools will be our ability to keep some interpretive control of the entire imaging process.

Biography
Samuel H. Gray received a BS (1970) from Georgetown University and a PhD (1978) in mathematics from the University of Denver. Between 1978 and 1982 he worked at the US Naval Research Lab in Washington, DC, and was a faculty member at General Motors Institute (now Kettering University) in Flint, MI. From 1982 to 1999 he worked for Amoco and bp, first in Amoco's Tulsa Research Center and later at Amoco Canada. While at Amoco and bp, he was a member of several teams building and using depth migration and velocity estimation tools. Since 1999, he has been at Veritas DGC, where he continues to work on depth imaging, velocity estimation, and seismic modeling techniques. He has served as Associate Editor of Geophysics, and he has co-authored papers which have won the Best Paper in Geophysics award (1999) and Honorable Mention for Best Paper in Geophysics (1998 and 2001).
James Sun received a PhD (1980) in Physics from University of California, Riverside. He spent two years doing postdoctoral research in atomic scattering theory before joining ARCO in 1982. He was a Research Advisor in ARCO's exploration research group until ARCO merged with BP. He joined Veritas in 2000 and is currently a Senior Research Scientist at Veritas Research in Houston.





